Differentiate between the following ( 2 x 10)
Chain line and check line.
Check Lines. The line which is run in the field to check the accuracy of the fieldwork is called the check line. If the measured length of a check line agrees with the length scaled off the plan, the survey is accurate.
Each triangle is generally provided with a check line. The check lines may be laid in such a way that a maximum number of details are intersected by it. Check lines may also be laid by joining the apex of the main triangle to any point on the opposite side or by joining two points on any two sides of the triangle.
Vernier scale and the chord scale
Vernier Scale A device used for measuring the fractional part of one of the smallest divisions of a graduated scale. It usually consists of a small auxiliary scale that slides alongside the main scale.
The least count of the vernier = the difference between the smallest division on the main division and the smallest division on the vernier scale.
Chord scale A scale of chords is used to measure or to set off angles. It is marked either on a rectangular protractor or on an ordinary box wooden scale.
Systematic errors and accidental errors.
- Systematic errors These are the errors that occur from well-understood causes and can be reduced by adopting suitable methods. For example, the error due to the sag of a tape supported at the ends can be calculated and subtracted from each measurement. However, the tape can be supported throughout its length at short intervals and the sag error may be reduced to a negligible quantity. It always has the same magnitude and signs so long as the conditions remain the same and such an error is called constant systematic error. Whereas, if the conditions change, the magnitude of the error changes and this is known as variable systematic error. A systematic error follows a definite mathematical or physical law and, therefore, a correction can always be determined and applied. lt| is also known as cumulative error.
- Accidental errors These are errors due to a combination of causes and are beyond the control of the surveyor. It can be a plus or minus. The calibration of a chain is an example of an accidental error.
Scale graduations in a prismatic compass and a surveyor compass.
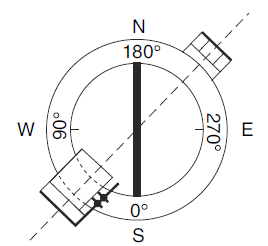
Prismatic Compass The ring is graduated from the south end of the needle. The observations run clockwise round to 360° with zero placed at the south. The graduations have 0° at S, 90° at W, 180° at N and 270° at E.
Surveyor Compass The 0° is placed in both north and south directions and 90° is marked in east and west directions. The graduations have 0° at N and S and 90° at E and W. The letters E and W are interchanged from their true positions in order to read the bearing in its proper quadrant.
Plate level test and spire test in theodolite.
Plate bubble test It is used to make the plate bubbles central to their run when the vertical axis of the theodolite is truly vertical When in perfect adjustment, the plate bubbles once centred remains central for all positions of the horizontal plate. If either or both the bubbles are not in the centre of their run, then an adjustment is required. The instrument is levelled as described in Section 4.8. The telescope is swing through 180° about the vertical axis, i.e., swing the telescope. If the bubble runs out of the centre, the adjustment is not in order. If it is so, count the number of graduations on the bubble tube by which the bubble has run out of its centre.
Spire test It is used to make the horizontal axis perpendicular to the vertical axis. The theodolite is set up and carefully levelled at about 50 m from a high building. A point, say A, on the building is bisected, preferably with a vertical angle greater than 30°. The telescope is then lowered and a point B is set near the ground. The face is changed and point A is sighted again. The telescope is then depressed. If the instrument is in adjustment, the line of sight will fall on the ground point vertically below point A set previously. If not, a second point B₂ is set near the ground. If e is the error in adjustment, then B₁B₂ will be equal to 2e.
Stadia tacheometry and subtense bar tacheometry.
Stadia system When the parallactic angle a, defined by means of stadia wires, is kept fixed and the staff intercept is varied, e.g., AB and A'B', the method is called the fixed hair method. A tachometer and a staff are used to make the observations.
Subtense bar tacheometry Another way can be to keep the staff intercept fixed, e.g., AB and A"B", and varying the parallactic angle, e.g., a and a'. The stadia wires will have to be moved and is accordingly known as the movable hair or subtense method. A subtense theodolite in conjunction with a staff or subtense bar is used to make the observations.
Tracing paper method and trial and error method in plane table surveying.
Tracing Paper Method
Procedure Setup the plane table at T. Fix a tracing paper on the plane table and transfer approximately station T as t on tracing paper (Fig. 8.14). Pivot the alidade against t. Sight stations A, B and C and draw rays towards each of them. Remove the tracing paper and move it on the drawing sheet in such a way that the three rays on the tracing paper pass through the plotted positions of the ground stations. Prick the point t on the drawing sheet. This gives the required position of the plane table. Remove the tracing paper. Place the alidade along ta and orient the table by turning it until the line of sight bisects A. Keep the alidade against b and draw a back ray. Keep the alidade such that it touches C and draw a back ray. These two rays should pass through t. However, if a triangle of error is formed because of careless manipulation, it can be eliminated by the trial-and-error method.
Trial & error methodCompound curve and transition curve.
Compound Curve
When two or more simple circular curves, of different radii, turning in the same direction join two intersecting straights, the resultant curve is known as a compound curve. In the figure, T₁TT₂ is a compound curve with two simple circular curves T₁T and TT₂ of radii R₁ and R₂, respectively. ATB is the common tangent, and T is the common tangent point.
Transition Curve
It is a curve usually introduced between a simple circular curve and a straight, or between two
simple circular curves. It is also known as an easement curve. A transition curve has a radius, gradually changing from a finite to an infinite value or vice versa. It is widely used on highways and railways, since its radius increases or decreases in a very gradual manner. In Fig., T₁TD is the transition curve introduced between the simple circular curve T₄DD' T₅ and the straight T₁I.
Triangulation and Trilateration.
Triangulation
When the area to be surveyed is of a considerable extent, triangulation is adopted. The entire area is divided into a network of triangles. Anyone side of any of the triangles so formed, is selected and is measured precisely. Such a line is called a baseline. All the angles in the network are measured with a transit. The lengths of the sides of all the triangles are then computed, from the measured length of the baseline and the observed corrected angles, using the sine formula.
Trilateration
Global Positioning System (GPS) is a satellite navigation system based on the principle of trilateration. Trilateration is a method used to find the location of a point if its distances from at least three other stations of known coordinates are predetermined.
Condition equation and Normal equation.
Condition equation
An equation that expresses exactly certain relationships that must exist among related quantities. For example: In measuring the angles of a triangle, no condition exists until all three angles are measured. The condition equation will then express the condition that the three measured angles plus certain corrections must equal 180° plus the spherical excess of the triangle. The various condition equations set up in surveying are—angle equation, side equation, length equation, latitude equation, longitude equation, and azimuth equation.
Normal (observation) equation
An ‘observation equation’ is the symbolic equality of a quantity and its observed value, e.g., ∠A = 145°32'20'' , or ∠B + ∠C = 67°35'17''. The former value is observed by direct single measurement and the latter one is observed indirectly when simultaneous observations or conditions are involved.
---
- The study material for AMIE/B Tech/Junior Engineer exams is available at https://amiestudycircle.com
- If you like the post please share your thoughts in the comment section










Comments